If one breaks down the visual field into its basic components one eventually arrives at the atom of vision, which is 'The Dot'. The visual system processes all the dots in its field and creates strings of dots which are recognized as lines, and if such a line is extended at 90 degrees to its own axis it becomes a 2-dimensional plane.

THE BUILDING BLOCKS OF VISUAL PERCEPTION

FIG 1:

STARTING WITH DOTS AS THE FUNDEMENTAL TYPE, THEY ARE . ESSENTIALLY SYMBOLIC OF THE 0-DIMENSION AND ARE
     REGARDED AS CORRUPT 0-DIMENSIONAL LINES SINCE THEY ARE VISIBLE AND THEREFORE NOT DIMENSIONLESS.

THE DOTS THEN UNDERGO EXTENSION ALONG THE HORIZONTAL TO BECOME SYMBOLIC Of 1-DIMENSlONAL LINES. THESE T00 ARE SEEN AS CORRUPT SINCE THEY ARE VISIBLE AND UNDER THE MICROSCOPE THEY APPEAR AS 2-DIMENSIONAL PLANES. ITMUST BE REMEMBERED THAT THE CONCERN IS THE NATURE OF THE IMAGE THAT FALLS UPON THE RETINA.

THE LINES THEN UNDERGO A VERTICAL EXTENSION TO BECOME SYMBOLIC, CORRUPT 2-DIMENSIONAL PLANES.
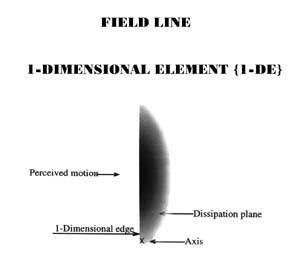
    A TRUE 1-DIMENSlONAL LINE OCCURS AS AN EDGE WHOSE VERTICAL EXTENSION RESLULTS IN THE DISSPATION OF THE LINEINTO THE BACKGROUND.

THE ACTUAL 1-DIMENSlONAL LINE IS WHERE THE EDGE OF IT'S ASSOCIATED (DISSPATING) PLANE MEETS THE PLANE OF
    THE BACKGROUND AND NOT WHERE IT FADES AWAY. (See Fig 2 Below)
 

A plane with a black surround, however, is not a real plane it is a symbol for a plane but there is a type of line that exists in nature that can be called a 'molecule' of vision. It is the 1-dimensional line [fig.l]. It is rather like a shadow with only one edge. It is the intersection of two infinitely large 2-dimensional planes and it is not a symbolic object.

This 1-dimensional object was invented by me in mid-1984 as the result of a similar analysis that was concerned in energizing the plane of a 2-dimensional surface with a single type of element. The notion was to build 'energized' surfaces by polarizing an array of such elements in such a way that used the idiosyncrasies of visual perception to create illusions of warped space while at the same time appealing to the elegance of simplicity that physicists and mathematicians so often speak of. The first thing was to organize the 2-D surface to take 1-D elements in an ordered way and for this the surface was made into a grid of about 25 x 25 square units. The corners of each unit represented an axis point to which a 1-D element was hinged. The 'elements' could then take up any orientation about the hinge axis. (See Field Lines)
FIG 2:
 
 
 

• The essence of a 1-D element (1-DE ) is that it possesses a single "edge" and That it be either negative or positive with respect to the background.
• Its associated 1-Dimensional line (edge) is neither negative nor positive.
• All other properties of the 1-DE pertain to the perception of it.

• For us to see a 1-DE it must exist either on the 2-D plane or the 3-D plane It can however still exist on the 1-D plane but this must be as an abstraction.

• It must differ in some way from the background plane and for our visual purposes that difference is one of colour or contrast..

FIG 3:

• The image above is a 1-DE as can be seen, it has a single hard edge of infinite length and is described by a plane which merges eventually into the background.

• The properties of this plane can be described as a fourier series

Projects Index
 
 

marek@panetix.com